NCERT Solutions for Class 8 Maths Chapter 3 Understanding Quadrilaterals are provided here, which can be downloaded for free in PDF format. The NCERT Solutions for the chapter Understanding Quadrilaterals are prepared by the mathematics experts at BYJU’S, from an examination point of view. These solutions explain the accurate method of solving problems. By understanding the concepts used in NCERT Solutions for Class 8, students will be able to clear all their doubts related to Understanding Quadrilaterals.
Download Exclusively Curated Chapter Notes for Class 8 Maths Chapter – 3 Understanding Quadrilaterals
Download Most Important Questions for Class 8 Maths Chapter – 3 Understanding Quadrilaterals
NCERT Solutions for Class 8 Maths Chapter 3 – Understanding Quadrilaterals
Access Answers of Maths NCERT Class 8 Chapter 3 – Understanding Quadrilaterals
Exercise 3.1 Page: 41
1. Given here are some figures.

Classify each of them on the basis of the following.
Simple curve (b) Simple closed curve (c) Polygon
(d) Convex polygon (e) Concave polygon
Solution:
a) Simple curve: 1, 2, 5, 6 and 7
b) Simple closed curve: 1, 2, 5, 6 and 7
c) Polygon: 1 and 2
d) Convex polygon: 2
e) Concave polygon: 1
2. How many diagonals does each of the following have?
a) A convex quadrilateral (b) A regular hexagon (c) A triangle
Solution:
a) A convex quadrilateral: 2.

b) A regular hexagon: 9.

c) A triangle: 0

3. What is the sum of the measures of the angles of a convex quadrilateral? Will this property hold if the quadrilateral is not convex? (Make a non-convex quadrilateral and try!)
Solution:

Let ABCD be a convex quadrilateral.
From the figure, we infer that the quadrilateral ABCD is formed by two triangles,
i.e. ΔADC and ΔABC.
Since we know that sum of the interior angles of a triangle is 180°,
the sum of the measures of the angles is 180° + 180° = 360°

Let us take another quadrilateral ABCD which is not convex .
Join BC, such that it divides ABCD into two triangles ΔABC and ΔBCD. In ΔABC,
∠1 + ∠2 + ∠3 = 180° (angle sum property of triangle)
In ΔBCD,
∠4 + ∠5 + ∠6 = 180° (angle sum property of triangle)
∴, ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 = 180° + 180°
⇒ ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 = 360°
⇒ ∠A + ∠B + ∠C + ∠D = 360°
Thus, this property holds if the quadrilateral is not convex.
4. Examine the table. (Each figure is divided into triangles and the sum of the angles deduced from that.)

What can you say about the angle sum of a convex polygon with number of sides? (a) 7 (b) 8 (c) 10 (d) n
Solution:
The angle sum of a polygon having side n = (n-2)×180°
a) 7
Here, n = 7
Thus, angle sum = (7-2)×180° = 5×180° = 900°
b) 8
Here, n = 8
Thus, angle sum = (8-2)×180° = 6×180° = 1080°
c) 10
Here, n = 10
Thus, angle sum = (10-2)×180° = 8×180° = 1440°
d) n
Here, n = n
Thus, angle sum = (n-2)×180°
5. What is a regular polygon?
State the name of a regular polygon of
(i) 3 sides (ii) 4 sides (iii) 6 sides
Solution:
Regular polygon: A polygon having sides of equal length and angles of equal measures is called a regular polygon. A regular polygon is both equilateral and equiangular.
(i) A regular polygon of 3 sides is called an equilateral triangle.
(ii) A regular polygon of 4 sides is called a square.
(iii) A regular polygon of 6 sides is called a regular hexagon.
6. Find the angle measure of x in the following figures.

Solution:
a) The figure has 4 sides. Hence, it is a quadrilateral. Sum of angles of the quadrilateral = 360°
⇒ 50° + 130° + 120° + x = 360°
⇒ 300° + x = 360°
⇒ x = 360° – 300° = 60°
b) The figure has 4 sides. Hence, it is a quadrilateral. Also, one side is perpendicular forming a right angle.
Sum of angles of the quadrilateral = 360°
⇒ 90° + 70° + 60° + x = 360°
⇒ 220° + x = 360°
⇒ x = 360° – 220° = 140°
c) The figure has 5 sides. Hence, it is a pentagon.

Sum of angles of the pentagon = 540° Two angles at the bottom are a linear pair.
∴, 180° – 70° = 110°
180° – 60° = 120°
⇒ 30° + 110° + 120° + x + x = 540°
⇒ 260° + 2x = 540°
⇒ 2x = 540° – 260° = 280°
⇒ 2x = 280°
= 140°
d) The figure has 5 equal sides. Hence, it is a regular pentagon. Thus, all its angles are equal.
5x = 540°
⇒ x = 540°/5
⇒ x = 108°
7.

Solution:
a) Sum of all angles of triangle = 180°
One side of triangle = 180°- (90° + 30°) = 60°
x + 90° = 180° ⇒ x = 180° – 90° = 90°
y + 60° = 180° ⇒ y = 180° – 60° = 120°
z + 30° = 180° ⇒ z = 180° – 30° = 150°
x + y + z = 90° + 120° + 150° = 360°
b) Sum of all angles of quadrilateral = 360°
One side of quadrilateral = 360°- (60° + 80° + 120°) = 360° – 260° = 100°
x + 120° = 180° ⇒ x = 180° – 120° = 60°
y + 80° = 180° ⇒ y = 180° – 80° = 100°
z + 60° = 180° ⇒ z = 180° – 60° = 120°
w + 100° = 180° ⇒ w = 180° – 100° = 80°
x + y + z + w = 60° + 100° + 120° + 80° = 360°
Exercise 3.2 Page: 44
1. Find x in the following figures.

Solution:
a)

125° + m = 180° ⇒ m = 180° – 125° = 55° (Linear pair)
125° + n = 180° ⇒ n = 180° – 125° = 55° (Linear pair)
x = m + n (The exterior angle of a triangle is equal to the sum of the two opposite interior angles)
⇒ x = 55° + 55° = 110°
b)

Two interior angles are right angles = 90°
70° + m = 180° ⇒ m = 180° – 70° = 110° (Linear pair)
60° + n = 180° ⇒ n = 180° – 60° = 120° (Linear pair) The figure is having five sides and is a pentagon.
Thus, sum of the angles of a pentagon = 540°
⇒ 90° + 90° + 110° + 120° + y = 540°
⇒ 410° + y = 540° ⇒ y = 540° – 410° = 130°
x + y = 180° (Linear pair)
⇒ x + 130° = 180°
⇒ x = 180° – 130° = 50°
2. Find the measure of each exterior angle of a regular polygon of
(i) 9 sides (ii) 15 sides
Solution:
Sum of the angles of a regular polygon having side n = (n-2)×180°
(i) Sum of the angles of a regular polygon having 9 sides = (9-2)×180°= 7×180° = 1260°
Each interior angle=1260/9 = 140°
Each exterior angle = 180° – 140° = 40°
Or,
Each exterior angle = Sum of exterior angles/Number of angles = 360/9 = 40°
(ii) Sum of angles of a regular polygon having side 15 = (15-2)×180°
= 13×180° = 2340°
Each interior angle = 2340/15 = 156°
Each exterior angle = 180° – 156° = 24°
Or,
Each exterior angle = sum of exterior angles/Number of angles = 360/15 = 24°
3. How many sides does a regular polygon have if the measure of an exterior angle is 24°?
Solution:
Each exterior angle = sum of exterior angles/Number of angles
24°= 360/ Number of sides
⇒ Number of sides = 360/24 = 15
Thus, the regular polygon has 15 sides.
4. How many sides does a regular polygon have if each of its interior angles is 165°?
Solution:
Interior angle = 165°
Exterior angle = 180° – 165° = 15°
Number of sides = sum of exterior angles/exterior angles
⇒ Number of sides = 360/15 = 24
Thus, the regular polygon has 24 sides.
5. a) Is it possible to have a regular polygon with measure of each exterior angle as 22°?
b) Can it be an interior angle of a regular polygon? Why?
Solution:
a) Exterior angle = 22°
Number of sides = sum of exterior angles/ exterior angle
⇒ Number of sides = 360/22 = 16.36
No, we can’t have a regular polygon with each exterior angle as 22° as it is not a divisor of 360.
b) Interior angle = 22°
Exterior angle = 180° – 22°= 158°
No, we can’t have a regular polygon with each exterior angle as 158° as it is not a divisor of 360.
6. a) What is the minimum interior angle possible for a regular polygon? Why?
b) What is the maximum exterior angle possible for a regular polygon?
Solution:
a) An equilateral triangle is the regular polygon (with 3 sides) having the least possible minimum interior angle because a regular polygon can be constructed with minimum 3 sides.
Since the sum of interior angles of a triangle = 180°
Each interior angle = 180/3 = 60°
b) An equilateral triangle is the regular polygon (with 3 sides) having the maximum exterior angle because the regular polygon with the least number of sides has the maximum exterior angle possible. Maximum exterior possible = 180 – 60° = 120°
Exercise 3.3 Page: 50
1. Given a parallelogram ABCD. Complete each statement along with the definition or property used.

(i) AD = …… (ii) ∠DCB = ……
(iii) OC = …… (iv) m ∠DAB + m ∠CDA = ……
Solution:
(i) AD = BC (Opposite sides of a parallelogram are equal)
(ii) ∠DCB = ∠DAB (Opposite angles of a parallelogram are equal)
(iii) OC = OA (Diagonals of a parallelogram are equal)
(iv) m ∠DAB + m ∠CDA = 180°
2. Consider the following parallelograms. Find the values of the unknown x, y, z

Solution:
(i)

y = 100° (opposite angles of a parallelogram)
x + 100° = 180° (adjacent angles of a parallelogram)
⇒ x = 180° – 100° = 80°
x = z = 80° (opposite angles of a parallelogram)
∴, x = 80°, y = 100° and z = 80°
 (ii)
(ii)
50° + x = 180° ⇒ x = 180° – 50° = 130° (adjacent angles of a parallelogram) x = y = 130° (opposite angles of a parallelogram)
x = z = 130° (corresponding angle)
(iii)

x = 90° (vertical opposite angles)
x + y + 30° = 180° (angle sum property of a triangle)
⇒ 90° + y + 30° = 180°
⇒ y = 180° – 120° = 60°
also, y = z = 60° (alternate angles)
(iv)

z = 80° (corresponding angle) z = y = 80° (alternate angles) x + y = 180° (adjacent angles)
⇒ x + 80° = 180° ⇒ x = 180° – 80° = 100°
(v)

x=28o
y = 112o z = 28o
3. Can a quadrilateral ABCD be a parallelogram if (i) ∠D + ∠B = 180°?
(ii) AB = DC = 8 cm, AD = 4 cm and BC = 4.4 cm?
(iii)∠A = 70° and ∠C = 65°?
Solution:
(i) Yes, a quadrilateral ABCD can be a parallelogram if ∠D + ∠B = 180° but it should also fulfil some conditions, which are:
(a) The sum of the adjacent angles should be 180°.
(b) Opposite angles must be equal.
(ii) No, opposite sides should be of the same length. Here, AD ≠ BC
(iii) No, opposite angles should be of the same measures. ∠A ≠ ∠C
4. Draw a rough figure of a quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measure.
Solution:

ABCD is a figure of quadrilateral that is not a parallelogram but has exactly two opposite angles, that is, ∠B = ∠D of equal measure. It is not a parallelogram because ∠A ≠ ∠C.
5. The measures of two adjacent angles of a parallelogram are in the ratio 3 : 2. Find the measure of each of the angles of the parallelogram.
Solution:
Let the measures of two adjacent angles ∠A and ∠B be 3x and 2x, respectively in
parallelogram ABCD.
∠A + ∠B = 180°
⇒ 3x + 2x = 180°
⇒ 5x = 180°
⇒ x = 36°
We know that opposite sides of a parallelogram are equal.
∠A = ∠C = 3x = 3 × 36° = 108°
∠B = ∠D = 2x = 2 × 36° = 72°
6. Two adjacent angles of a parallelogram have equal measure. Find the measure of each of the angles of the parallelogram.
Solution:
Let ABCD be a parallelogram.
Sum of adjacent angles of a parallelogram = 180°
∠A + ∠B = 180°
⇒ 2∠A = 180°
⇒ ∠A = 90°
also, 90° + ∠B = 180°
⇒ ∠B = 180° – 90° = 90°
∠A = ∠C = 90°
∠B = ∠D = 90
°
7. The adjacent figure HOPE is a parallelogram. Find the angle measures x, y and z. State the properties you use to find them.

Solution:
y = 40° (alternate interior angle)
∠P = 70° (alternate interior angle)
∠P = ∠H = 70° (opposite angles of a parallelogram)
z = ∠H – 40°= 70° – 40° = 30°
∠H + x = 180°
⇒ 70° + x = 180°
⇒ x = 180° – 70° = 110°
8. The following figures GUNS and RUNS are parallelograms. Find x and y. (Lengths are in cm)

Solution:
(i) SG = NU and SN = GU (opposite sides of a parallelogram are equal) 3x = 18
x = 18/3
⇒ x =6
3y – 1 = 26
⇒ 3y = 26 + 1
⇒ y = 27/3=9
x = 6 and y = 9
(ii) 20 = y + 7 and 16 = x + y (diagonals of a parallelogram bisect each other) y + 7 = 20
⇒ y = 20 – 7 = 13 and,
x + y = 16
⇒ x + 13 = 16
⇒ x = 16 – 13 = 3
x = 3 and y = 13
9. In the above figure both RISK and CLUE are parallelograms. Find the value of x.

Solution:
∠K + ∠R = 180° (adjacent angles of a parallelogram are supplementary)
⇒ 120° + ∠R = 180°
⇒ ∠R = 180° – 120° = 60°
also, ∠R = ∠SIL (corresponding angles)
⇒ ∠SIL = 60°
also, ∠ECR = ∠L = 70° (corresponding angles) x + 60° + 70° = 180° (angle sum of a triangle)
⇒ x + 130° = 180°
⇒ x = 180° – 130° = 50°
10. Explain how this figure is a trapezium. Which of its two sides are parallel? (Fig 3.32)

Solution:
When a transversal line intersects two lines in such a way that the sum of the adjacent angles on the same side of transversal is 180°, then the lines are parallel to each other. Here, ∠M + ∠L = 100° + 80° = 180°
Thus, MN || LK
As the quadrilateral KLMN has one pair of parallel lines, it is a trapezium. MN and LK are parallel lines.
11. Find m∠C in Fig 3.33 if AB || DC.

Solution:
m∠C + m∠B = 180° (angles on the same side of transversal)
⇒ m∠C + 120° = 180°
⇒ m∠C = 180°- 120° = 60°
12. Find the measure of ∠P and ∠S if SP || RQ ? in Fig 3.34. (If you find m∠R, is there more than one method to find m∠P?)

Solution:
∠P + ∠Q = 180° (angles on the same side of transversal)
⇒ ∠P + 130° = 180°
⇒ ∠P = 180° – 130° = 50°
also, ∠R + ∠S = 180° (angles on the same side of transversal)
⇒ 90° + ∠S = 180°
⇒ ∠S = 180° – 90° = 90°
Thus, ∠P = 50° and ∠S = 90°
Yes, there are more than one method to find m∠P.
PQRS is a quadrilateral. Sum of measures of all angles is 360°.
Since, we know the measurement of ∠Q, ∠R and ∠S.
∠Q = 130°, ∠R = 90° and ∠S = 90°
∠P + 130° + 90° + 90° = 360°
⇒ ∠P + 310° = 360°
⇒ ∠P = 360° – 310° = 50°
Exercise 3.4 Page: 55
1. State whether True or False.
(a) All rectangles are squares.
(b) All rhombuses are parallelograms.
(c) All squares are rhombuses and also rectangles.
(d) All squares are not parallelograms.
(e) All kites are rhombuses.
(f) All rhombuses are kites.
(g) All parallelograms are trapeziums.
(h) All squares are trapeziums.
Solution:
(a) False
Because all squares are rectangles but all rectangles are not squares.
(b) True
(c) True
(d) False
Because all squares are parallelograms as opposite sides are parallel and opposite angles are equal.
(e) False.
Because, for example, the length of the sides of a kite are not of the same length.
(f) True
(g) True
(h) True
2. Identify all the quadrilaterals that have.
(a) four sides of equal length (b) four right angles
Solution:
(a) Rhombus and square have all four sides of equal length.
(b) Square and rectangle have four right angles.
3. Explain how a square is
(i) a quadrilateral (ii) a parallelogram (iii) a rhombus (iv) a rectangle
Solution
(i) Square is a quadrilateral because it has four sides.
(ii) Square is a parallelogram because it’s opposite sides are parallel and opposite angles are equal.
(iii) Square is a rhombus because all the four sides are of equal length and diagonals bisect at right angles.
(iv)Square is a rectangle because each interior angle, of the square, is 90°
4. Name the quadrilaterals whose diagonals.
(i) bisect each other (ii) are perpendicular bisectors of each other (iii) are equal
Solution
(i) Parallelogram, Rhombus, Square and Rectangle
(ii) Rhombus and Square
(iii)Rectangle and Square
5. Explain why a rectangle is a convex quadrilateral.
Solution
A rectangle is a convex quadrilateral because both of its diagonals lie inside the rectangle.
6. ABC is a right-angled triangle and O is the mid-point of the side opposite to the right angle. Explain why O is equidistant from A, B and C. (The dotted lines are drawn additionally to help you).
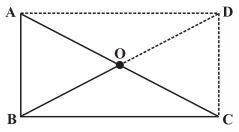
Solution
AD and DC are drawn so that AD || BC and AB || DC
AD = BC and AB = DC
ABCD is a rectangle as opposite sides are equal and parallel to each other and all the interior angles are of 90°.
In a rectangle, diagonals are of equal length and also bisect each other.
Hence, AO = OC = BO = OD
Thus, O is equidistant from A, B and C.
| Also Access |
| NCERT Exemplar for Class 8 Maths Chapter 3 |
| CBSE Notes for Class 8 Maths Chapter 3 |
NCERT Solutions for Class 8 Maths Chapter 3 – Understanding Quadrilaterals
The major concepts covered in this chapter include: 3.1 Introduction 3.2 Polygons 3.2.1 Classification of Polygons 3.2.2 Diagonals 3.2.3 Convex and Concave Polygons 3.2.4 Regular and Irregular Polygons 3.2.5 Angle sum property 3.3 Sum of the Measures of the Exterior Angles of a Polygon 3.4 Kinds of Quadrilaterals 3.4.1 Trapezium 3.4.2 Kite 3.4.3 Parallelogram 3.4.4 Elements of a parallelogram 3.4.5 Angles of parallelogram 3.4.6 Diagonals of a parallelogram 3.5 Some special parallelograms 3.5.1 Rhombus 3.5.2 A rectangle 3.5.3 A square
Exercise 3.1 Solutions 7 Questions (1 Long Answer Question, 6 Short Answer Questions)
Exercise 3.2 Solutions 6 Questions (6 Short Answer Questions)
Exercise 3.3 Solutions 12 Questions (6 Long Answer Questions, 6 Short Answer Questions)
Exercise 3.4 Solutions 6 Questions (1 Long Answer Question, 5 Short Answer Questions)
NCERT Solutions for Class 8 Maths Chapter 3 – Understanding Quadrilaterals
Chapter 3 of NCERT Solutions for Class 8 Maths helps you understand the fundamental concepts related to quadrilaterals. From explanations of quadrilaterals to different types of quadrilaterals, the chapter mainly discusses the following concepts:
1. Parallelogram: A quadrilateral with each pair of opposite sides parallel.
Properties: Opposite sides are equal. Opposite angles are equal. Diagonals bisect one another
2. Rhombus: A parallelogram with sides of equal length.
Properties: All the properties of a parallelogram. Diagonals are perpendicular to each other
3. Rectangle: A parallelogram with a right angle.
Properties: All the properties of a parallelogram. Each of the angles is a right angle. Diagonals are equal.
4. Square: A rectangle with sides of equal length.
Properties: All the properties of a parallelogram, rhombus and a rectangle.
5. Kite: A quadrilateral with exactly two pairs of equal consecutive sides
Properties: The diagonals are perpendicular to one another. One of the diagonals bisects the other.
Learning the chapter Understanding Quadrilaterals enables the students to understand the following:
-
- Properties of quadrilaterals: Sum of angles of a quadrilateral is equal to 360o
- Properties of parallelogram: Opposite sides of a parallelogram are equal. Opposite angles of a parallelogram are equal.
- Diagonals of a parallelogram bisect each other.
- Diagonals of a rectangle are equal and bisect each other.
- Diagonals of a rhombus bisect each other at right angles.
- Diagonals of a square are equal and bisect each other at right angles.
Disclaimer:
Dropped Topics – 3.1 Introduction, 3.2 Polygons, 3.2.1 Classification of polygons, 3.2.2 Diagonals and 3.2.5 Angle sum property.
Frequently Asked Questions on NCERT Solutions for Class 8 Maths Chapter 3
What kind of questions are there in NCERT Solutions for Class 8 Maths Chapter 3?
1. Exercise 3.1 with 7 Questions having 1 Long Answer Question, 6 Short Answer Questions
2. Exercise 3.2 with 6 Questions having 6 Short Answer Questions
3. Exercise 3.3 with 12 Questions having 6 Long Answer Questions, 6 Short Answer Questions
4. Exercise 3.4 with 6 Questions having 1 Long Answer Question, 5 Short Answer Questions

























it was so helpful for all
This is a very good app
It’s really very helpful app 👍👍
I like this app
This app help me so much
Thanks byjus for help to all students
Thank you for this app
It is so helpful for me and my father to explain me
This is a very helpful I loved it it’s good app