RD Sharma Solutions for Class 9 Maths Chapter 1 Number System

RD Sharma Solutions for Class 9 Maths Chapter 1 – Free PDF Download

RD Sharma Solutions for Class 9 Maths Chapter 1 Number System are given here to help students secure high marks in exams. Chapter 1 of Class 9 Maths mainly deals with problems based on rational and irrational numbers, natural numbers, whole numbers, representation of real numbers and many more. In order to strengthen their basic fundamentals of the number system, students must practise RD Sharma Solutions regularly. Students can access all chapters of RD Sharma Class 9 Solutions in both online and offline modes.

The RD Sharma textbook for Class 9 is based on the latest syllabus prescribed by the Central Board of Secondary Education (CBSE). In this textbook, at the end of each chapter, an exercise consisting of multiple-choice questions and a summary for quick revision of concepts and formulae have been given. In order to excel in the annual exams, it is suggested to refer to RD Sharma Solutions for Class 9 while practising textbook problems.

RD Sharma Solutions for Class 9 Maths Chapter 1 Number System

Download PDF

carouselExampleControls111

Previous



Next

Access Answers to RD Sharma Solutions for Class 9 Maths Chapter 1 Number System

Exercise 1.1

Question 1: Is zero a rational number? Can you write it in the form p/q, where p and q are integers and q ≠ 0?
Solution:

Yes, zero is a rational number.

It can be written in p/q form, provided that q ≠ 0.

For example, 0/1 or 0/3 or 0/4 etc.

Question 2: Find five rational numbers between 1 and 2.
Solution:

We know that one rational number between two numbers m and n = (m+n)/2

To find: 5 rational numbers between 1 and 2

Step 1: Rational number between 1 and 2

= (1+2)/2

= 3/2

Step 2: Rational number between 1 and 3/2

= (1+3/2)/2

= 5/4

Step 3: Rational number between 1 and 5/4

= (1+5/4)/2

= 9/8

Step 4: Rational number between 3/2 and 2

= 1/2 [(3/2) + 2)]

= 7/4

Step 5: Rational number between 7/4 and 2

= 1/2 [7/4 + 2]

= 15/8

Arrange all the results: 1 < 9/8 < 5/4 < 3/2 < 7/4 < 15/8 < 2

Therefore required integers are, 9/8, 5/4, 3/2, 7/4, 15/8

Question 3: Find six rational numbers between 3 and 4.

Solution:

Steps to find n rational numbers between any two numbers:

Step 1: Multiply and divide both the numbers by n+1.

In this example, we have to find 6 rational numbers between 3 and 4. Here n = 6

Multiply 3 and 4 by 7

3 x 7/7 = 21/7 and

4 x 7/7 = 28/7

Step 2: Choose 6 numbers between 21/7 and 28/7

3 = 21/7 < 22/7 < 23/7 < 24/7 < 25/7 < 26/7 < 27/7 < 28/7 = 4

Therefore, 6 rational numbers between 3 and 4 are

22/7, 23/7, 24/7, 25/7, 26/7, 27/7

Question 4: Find five rational numbers between 3/5 and 4/5.

Solution:

Steps to find n rational numbers between any two numbers:

Step 1: Multiply and divide both the numbers by n+1.

In this example, we have to find 5 rational numbers between 3/5 and 4/5. Here n = 5

Multiply 3/5 and 4/5 by 6

3/5 x 6/6 = 18/30 and

4/5 x 6/6 = 24/30

Step 2: Choose 5 numbers between 18/30 and 24/30

3/5 = 18/30 < 19/30 < 20/30 < 21/30 < 22/30 < 23/30 < 24/30 = 4/5

Therefore, 5 rational numbers between 3/5 and 4/5 are

19/30, 20/30, 21/30, 22/30, 23/30

Question 5: Are the following statements true or false? Give reasons for your answer.

(i) Every whole number is a natural number.

(ii) Every integer is a rational number.

(iii) Every rational number is an integer.

(iv) Every natural number is a whole number,

(v) Every integer is a whole number.

(vi) Every rational number is a whole number.

Solution:

(i) False.

Reason: As 0 is not a natural number.

(ii) True.

(iii) False.

Reason: Numbers such as 1/2, 3/2, and 5/3 are rational numbers but not integers.

(iv) True.

(v) False.

Reason: Negative numbers are not whole numbers.

(vi) False.

Reason: Proper fractions are not whole numbers.

Exercise 1.2

Question 1: Express the following rational numbers as decimals.
(i) 42/100 (ii) 327/500 (iii) 15/4

Solution:

Question 2: Express the following rational numbers as decimals.
(i) 2/3 (ii) -4/9 (iii) -2/15 (iv) -22/13 (v) 437/999 (vi) 33/26
Solution:

(i) Divide 2/3 using long division:

(ii) Divide using long division: -4/9

(iii) Divide using long division: -2/15

(iv) Divide using long division: -22/13

(v) Divide using long division: 437/999

(vi) Divide using long division: 33/26

Question 3: Look at several examples of rational numbers in the form p/q (q ≠ 0), where p and q are integers with no common factors other than 1 and having terminating decimal representations. Can you guess what property q must satisfy?

Solution:

The decimal representation will be terminating if the denominators have factors 2 or 5, or both. Therefore, p/q is a terminating decimal when the prime factorization of q must have only powers of 2 or 5 or both.

Exercise 1.3

Question 1: Express each of the following decimals in the form p/q:

(i) 0.39

(ii) 0.750

(iii) 2.15

(iv) 7.010

(v) 9.90

(vi) 1.0001

Solution:

(i)

0.39 = 39/100

(ii)

0.750 = 750/1000 = 3/4

(iii)

2.15 = 215/100 = 43/20

(iv)

7.010 = 7010/1000 = 701/100

(v)

9.90 = 990/100 = 99/10

(vi)

1.0001 = 10001/10000

Question 2: Express each of the following decimals in the form p/q:

Solution:

(i) Let x = 0.4̅

or x = 0.4̅ = 0.444 …. (1)

Multiplying both sides by 10

10x = 4.444 …..(2)

Subtract (1) by (2), and we get

10x – x = 4.444… – 0.444…

9x = 4

x = 4/9

=> 0.4̅ = 4.9

(ii) Let x = 0.3737.. …. (1)

Multiplying both sides by 100

100x = 37.37… …..(2)

Subtract (1) from (2), and we get

100x – x = 37.37… – 0.3737…

100x – x = 37

99x = 37

x = 37/99

(iii) Let x = 0.5454… (1)

Multiplying both sides by 100

100x = 54.5454…. (2)

Subtract (1) from (2), and we get

100x – x = 54.5454…. – 0.5454….

99x = 54

x = 54/99

(iv) Let x = 0.621621… (1)

Multiplying both sides by 1000

1000x = 621.621621…. (2)

Subtract (1) from (2), and we get

1000x – x = 621.621621…. – 0.621621….

999x = 621

x = 621/999

or x = 23/37

(v) Let x = 125.3333…. (1)

Multiplying both sides by 10

10x = 1253.3333…. (2)

Subtract (1) from (2), and we get

10x – x = 1253.3333…. – 125.3333….

9x = 1128

or x = 1128/9

or x = 376/3

(vi) Let x = 4.7777…. (1)

Multiplying both sides by 10

10x = 47.7777…. (2)

Subtract (1) from (2), and we get

10x – x = 47.7777…. – 4.7777….

9x = 43

x = 43/9

(vii) Let x = 0.47777….

Multiplying both sides by 10

10x = 4.7777…. …(1)

Multiplying both sides by 100

100x = 47.7777…. (2)

Subtract (1) from (2), and we get

100x – 10x = 47.7777…. – 4.7777…

90x = 43

x = 43/90

Exercise 1.4

Question 1: Define an irrational number.

Solution:

A number which cannot be expressed in the form of p/q, where p and q are integers and q ≠ 0. It is a non-terminating or non-repeating decimal.

Question 2: Explain how irrational numbers differ from rational numbers.

Solution:

An irrational number is a real number which can be written as a decimal but not as a fraction i.e. it cannot be expressed as a ratio of integers.

It cannot be expressed as terminating or repeating decimals.

For example, √2 is an irrational number

A rational number is a real number which can be written as a fraction, and as a decimal i.e. it can be expressed as a ratio of integers.

It can be expressed as a terminating or repeating decimal.

For example, 0.10 and 5/3 are rational numbers

Question 3: Examine whether the following numbers are rational or irrational:

Solution:

(i) √7

Not a perfect square root, so it is an irrational number.

(ii) √4

A perfect square root of 2.

We can express 2 in the form of 2/1, so it is a rational number.

(iii) 2 + √3

Here, 2 is a rational number, but √3 is an irrational number.

Therefore, the sum of a rational and irrational number is an irrational number.

(iv) √3 + √2

√3 is not a perfect square, thus an irrational number.

√2 is not a perfect square, thus an irrational number.

Therefore, the sum of √2 and √3 gives an irrational number.

(v) √3 + √5

√3 is not a perfect square, and hence, it is an irrational number

Similarly, √5 is not a perfect square, and it is an irrational number.

Since the sum of two irrational numbers is an irrational number, √3 + √5 is an irrational number.

(vi) (√2 – 2)²

(√2 – 2)² = 2 + 4 – 4 √2

= 6 – 4 √2

Here, 6 is a rational number but 4√2 is an irrational number.

Since the sum of a rational and an irrational number is an irrational number, (√2 – 2)2 is an irrational number.

(vii) (2 – √2)(2 + √2)

We can write the given expression as;

(2 – √2)(2 + √2) = ((2)² − (√2)²)

= 4 – 2 = 2 or 2/1

Since 2 is a rational number, (2 – √2)(2 + √2) is a rational number.

(viii) (√3 + √2)²

We can write the given expression as;

(√3 + √2)² = (√3)² + (√2)² + 2√3 x √2

= 3 + 2 + 2√6

= 5 + 2√6

Since the sum of a rational number and an irrational number is an irrational number, (√3 + √2)² is an irrational number.

(ix) √5 – 2

√5 is an irrational number, whereas 2 is a rational number.

The difference of an irrational number and a rational number is an irrational number.

Therefore, √5 – 2 is an irrational number.

(x) √23

Since, √23 = 4.795831352331…

As the decimal expansion of this number is non-terminating and non-recurring, it is an irrational number.

(xi) √225

√225 = 15 or 15/1

√225 is a rational number as it can be represented in the form of p/q, and q is not equal to zero.

(xii) 0.3796

As the decimal expansion of the given number is terminating, it is a rational number.

(xiii) 7.478478……

As the decimal expansion of this number is a non-terminating recurring decimal, it is a rational number.

(xiv) 1.101001000100001……

As the decimal expansion of the given number is non-terminating and non-recurring, it is an irrational number.

Question 4: Identify the following as rational or irrational numbers. Give the decimal representation of rational numbers:

Solution:

(i) √4

√4 = 2, which can be written in the form of a/b. Therefore, it is a rational number.

Its decimal representation is 2.0.

(ii) 3√18

3√18 = 9√2

Since the product of a rational and an irrational number is an irrational number.

Therefore, 3√18 is an irrational number.

Or 3 × √18 is an irrational number.

(iii) √1.44

√1.44 = 1.2

Since every terminating decimal is a rational number, √1.44 is a rational number.

And its decimal representation is 1.2.

(iv) √9/27

√9/27 = 1/√3

Since the quotient of a rational and an irrational number is irrational numbers, √9/27 is an irrational number.

(v) – √64

– √64 = – 8 or – 8/1

Therefore, – √64 is a rational number.

Its decimal representation is –8.0.

(vi) √100

√100 = 10

Since 10 can be expressed in the form of a/b, such as 10/1, √100 is a rational number.

And its decimal representation is 10.0.

Question 5: In the following equation, find which variables x, y, z etc. represent rational or irrational numbers:

Solution:

(i) x² = 5

Taking square root on both sides,

x = √5

√5 is not a perfect square root, so it is an irrational number.

(ii) y² = 9

y² = 9

or y = 3

3 can be expressed in the form of a/b, such as 3/1, so it is a rational number.

(iii) z² = 0.04

z² = 0.04

Taking square root on both sides, we get

z = 0.2

0.2 can be expressed in the form of a/b, such as 2/10, so it is a rational number.

(iv) u² = 17/4

Taking square root on both sides, we get

u = √17/2

Since the quotient of an irrational and a rational number is irrational, u is an Irrational number.

(v) v² = 3

Taking square root on both sides, we get

v = √3

Since √3 is not a perfect square root, so v is an irrational number.

(vi) w² = 27

Taking square root on both sides, we get

w = 3√3

Since the product of a rational and irrational is an irrational number, w is an irrational number.

(vii) t² = 0.4

Taking square root on both sides, we get

t = √(4/10)

t = 2/√10

Since the quotient of a rational and an irrational number is an irrational number, t is an irrational number.

Exercise 1.5

Question 1: Complete the following sentences:

(i) Every point on the number line corresponds to a …… number which may be either …… or …….

(ii) The decimal form of an irrational number is neither ….. nor ……

(iii) The decimal representation of a rational number is either ..… or …..

(iv) Every real number is either … number or … number.

Solution:

(i) Every point on the number line corresponds to a real number which may be either rational or irrational.

(ii) The decimal form of an irrational number is neither terminating nor repeating.

(iii) The decimal representation of a rational number is either terminating or non-terminating recurring.

(iv) Every real number is either a rational number or an irrational number.

Question 2: Represent √6, √7, √8 on the number line.

Solution:

Find the equivalent values of √6, √7, √8

√6 = 2.449

√7 = 2.645

√8 = 2.828

We can see that all the given numbers lie between 2 and 3.

Draw on the number line:

Question 3: Represent √3.5, √9.4, √10.5 and on the real number line.

Solution:

Represent √3.5 on the number line

Step 1: Draw a line segment AB = 3.5 units

Step 2: Produce B till point C, such that BC = 1 unit

Step 3: Find the mid-point of AC, say O.

Step 4: Taking O as the centre, draw a semi circle, passing through A and C.

Step 5: Draw a line passing through B perpendicular to OB, and cut a semicircle at D.

Step 6: Consider B as a centre and BD as the radius draw an arc cutting OC produced at E.

Now, from the right triangle OBD,

BD² = OD² – OB²

= OC² – (OC – BC)²

(As, OD = OC)

BD² = 2OC x BC – (BC)²

= 2 x 2.25 x 1 – 1

= 3.5

=> BD = √3.5

Represent √9.4 on the number line

Step 1: Draw a line segment AB = 9.4 units

Follow steps 2 to 6 mentioned above.

BD² = 2OC x BC – (BC)²

= 2 x 5.2 x 1 – 1

= 9.4

=> BD = √9.4

Represent √10.5 on the number line

Step 1: Draw a line segment AB = 10.5 units

Following the steps 2 to 6 mentioned above, we get

BD² = 2OC x BC – (BC)²

= 2 x 5.75 x 1 – 1

= 10.5

=> BD = √10.5

Question 4: Find whether the following statements are true or false:

(i) Every real number is either rational or irrational.

(ii) π is an irrational number.

(iii) Irrational numbers cannot be represented by points on the number line.

Solution:

(i) True.

(ii) True.

(ii) False.

Exercise 1.6

Question 1: Visualise 2.665 on the number line using successive magnification.

Solution:

2.665 lies between 2 and 3 on the number line.

Divide the selected segment into 10 equal parts and mark each point of division as 2.1, 2.2, ….,2.9, 2.10

2.665 lies between 2.6 and 2.7

Divide the line segment between 2.6 and 2.7 into 10 equal parts, such as 2.661, 2.662, and so on.

Here we can see that the 5th point will represent 2.665.

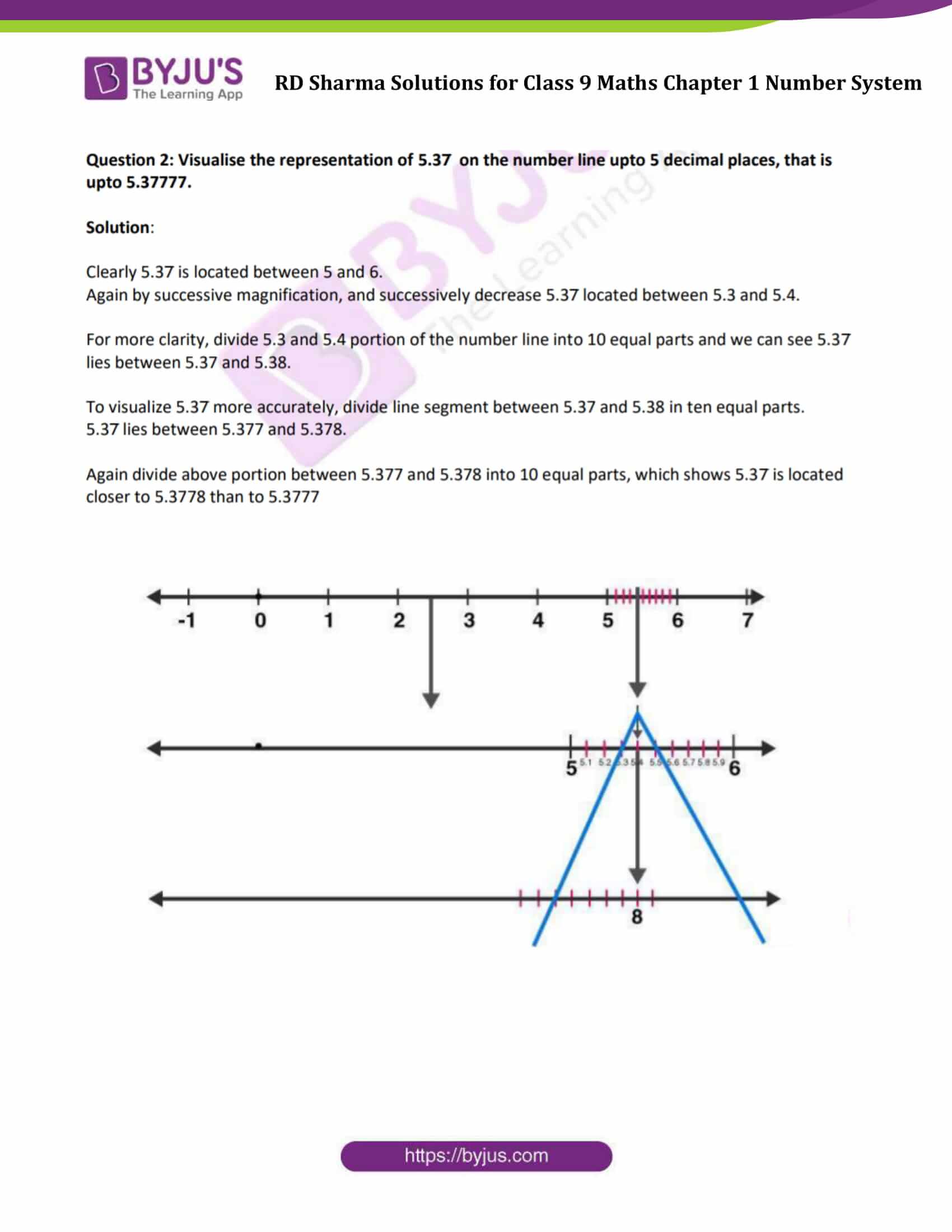
Question 2: Visualise the representation of 5.37̅ on the number line up to 5 decimal places, that is up to 5.37777.

Solution:

Clearly, 5.37̅ is located between 5 and 6.

Again by successive magnification, and successively decrease 5.37̅ located between 5.3 and 5.4.

For more clarity, divide the 5.3 and 5.4 portions of the number line into 10 equal parts, and we can see 5.37̅ lies between 5.37 and 5.38.

To visualize 5.37̅ more accurately, divide the line segment between 5.37 and 5.38 into ten equal parts.

5.37̅ lies between 5.377 and 5.378.

Again divide the above portion between 5.377 and 5.378 into 10 equal parts, which shows 5.37̅ is located closer to 5.3778 than to 5.3777

RD Sharma Solutions for Class 9 Maths Chapter 1 Number System

In the 1st chapter of Class 9 RD Sharma Solutions, students will study important concepts on the Number System as listed below:

• Number system introduction
• Review of numbers
• Decimal representation of rational numbers
• Conversion of decimal numbers into rational numbers
• Irrational numbers
• Some useful results on irrational numbers
• Representing irrational numbers on the number line
• Real numbers and real number line
• Existence of the square root of a positive real number
• Visualisation of representation of real numbers

Key Benefits of Studying RD Sharma Solutions for Class 9 Maths Chapter 1:

• The examples in RD Sharma Solutions are given in a step-by-step method for a comfortable and better understanding of concepts.
• The topics provided in the books are explained in simple and understandable language so that students can understand the concepts quickly.
• It also provides labelled diagrams and descriptive tables for effective study, which creates interest in learning.
• RD Sharma Solutions strictly follow the current CBSE syllabus and guidelines and provide a lot of questions to solve.