RD Sharma Solutions for Class 9 Maths Chapter 3 Rationalisation

RD Sharma Solutions for Class 9 Maths Chapter 3 – Free PDF Download

RD Sharma Solutions for Class 9 Maths Chapter 3 – Rationalisation is one of the most important chapters in Class 9 Maths. Students can learn about different algebraic identities and rationalisation of the denominator in RD Sharma Solutions for Class 9 Chapter 3. The rationalisation is a process by which radicals in the denominator of a fraction are eliminated. In this chapter, students will learn to simplify algebraic expressions using identities. Students are advised to memorise all the identities before they attempt any rationalisation question. To download the PDF of Chapter 3, click on the below link.

RD Sharma Solutions are useful for students as it helps them in scoring high marks in the annual examination. These solutions are prepared by subject-matter experts at BYJU’S, describing the complete method of solving problems. In order to excel in exams, students can access and download the PDF of RD Sharma Solutions for Class 9 Maths Chapter 3.

RD Sharma Solutions for Class 9 Maths Chapter 3 Rationalisation

Download PDF

carouselExampleControls111

Previous



Next

Access Answers to RD Sharma Solutions for Class 9 Maths Chapter 3 Rationalisation

Exercise 3.1

Question 1: Simplify each of the following:

Solution:

(i)

(ii)

Question 2: Simplify the following expressions:

(i) (4 + √7) (3 + √2)

(ii) (3 + √3)(5- √2 )

(iii) (√5 -2)( √3 – √5)

Solution:

(i) (4 + √7) (3 + √2)

= 12 + 4√2 + 3√7 + √14

(ii) (3 + √3)(5- √2 )

= 15 – 3√2 + 5√3 – √6

(iii) (√5 -2)( √3 – √5)

= √15 – √25 – 2√3 + 2√5

= √15 – 5 – 2√3 + 2√5

Question 3: Simplify the following expressions:

(i) (11 + √11) (11 – √11)

(ii) (5 + √7) (5 –√7 )

(iii) (√8 – √2 ) (√8 + √2 )

(iv) (3 + √3) (3 – √3)

(v) (√5 – √2) (√5 + √2)

Solution:

Using Identity: (a – b)(a+b) = a² – b²

(i) (11 + √11) (11 – √11)

= 11² – (√11)²

= 121 – 11

= 110

(ii) (5 + √7) (5 –√7 )

= (5² – (√7)² )

= 25 – 7 = 18

(iii) (√8 – √2 ) (√8 + √2 )

= (√8)² – (√2 ) ²

= 8 -2

= 6

(iv) (3 + √3) (3 – √3)

= (3)² – (√3)²

= 9 – 3

= 6

(v) (√5 – √2) (√5 + √2)

=(√5)² – (√2)²

= 5 – 2

= 3

Question 4: Simplify the following expressions:

(i) (√3 + √7)²

(ii) (√5 – √3)²

(iii) (2√5 + 3√2 )²

Solution:

Using identities: (a – b)² = a² + b² – 2ab and (a + b)² = a² + b² + 2ab

(i) (√3 + √7)²

= (√3)² + (√7)² + 2(√3)( √7)

= 3 + 7 + 2√21

= 10 + 2√21

(ii) (√5 – √3)²

= (√5)² + (√3)² – 2(√5)( √3)

= 5 + 3 – 2√15

= 8 – 2√15

(iii) (2√5 + 3√2 )²

= (2√5)² + (3√2 )² + 2(2√5 )( 3√2)

= 20 + 18 + 12√10

= 38 + 12√10

Exercise 3.2

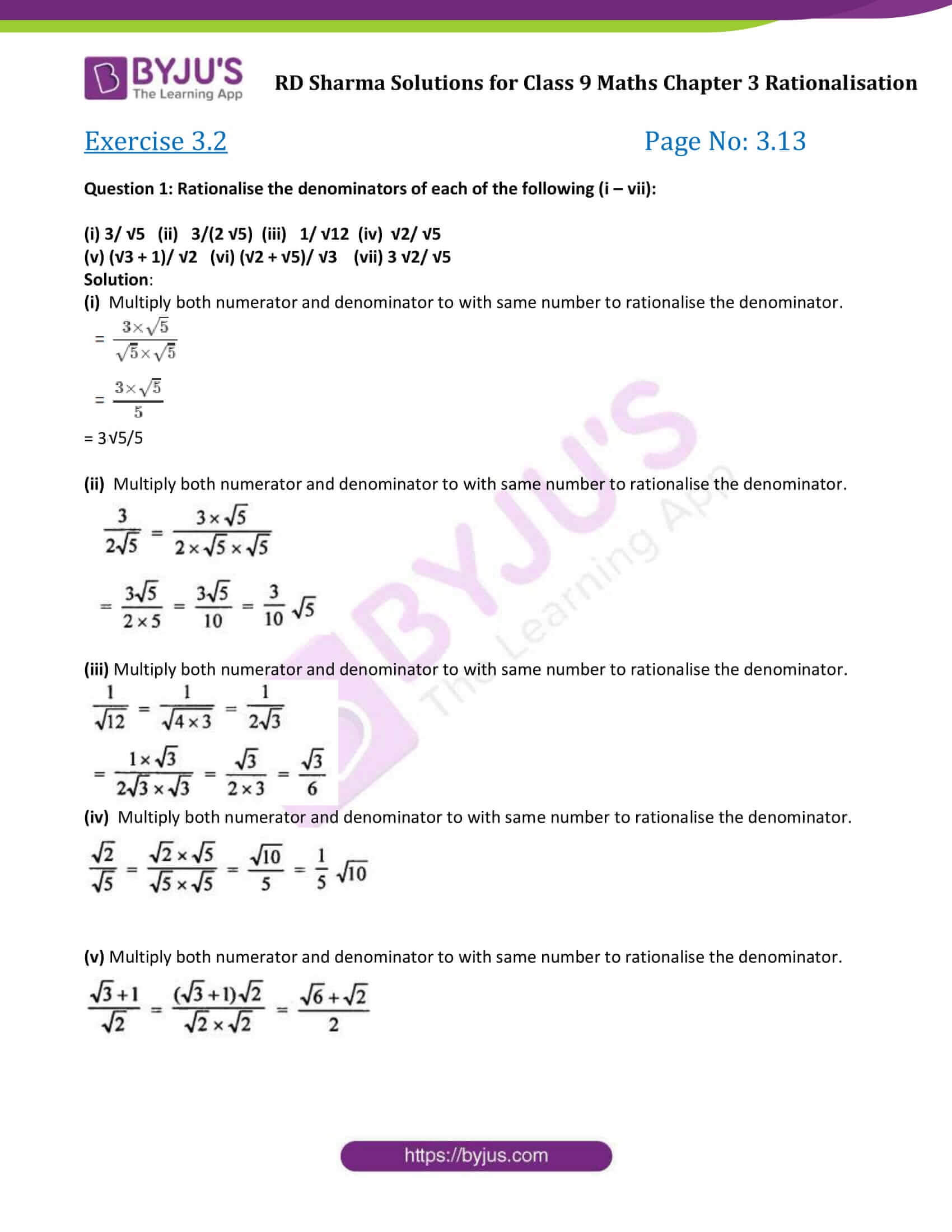
Question 1: Rationalise the denominators of each of the following (i – vii):

(i) 3/ √5 (ii) 3/(2 √5) (iii) 1/ √12 (iv) √2/ √5

(v) (√3 + 1)/ √2 (vi) (√2 + √5)/ √3 (vii) 3 √2/ √5

Solution:

(i) Multiply both the numerator and denominator with the same number to rationalise the denominator.

= 3√5/5

(ii) Multiply both the numerator and denominator with the same number to rationalise the denominator.

(iii) Multiply both the numerator and denominator with the same number to rationalise the denominator.

(iv) Multiply both the numerator and denominator with the same number to rationalise the denominator.

(v) Multiply both the numerator and denominator with the same number to rationalise the denominator.

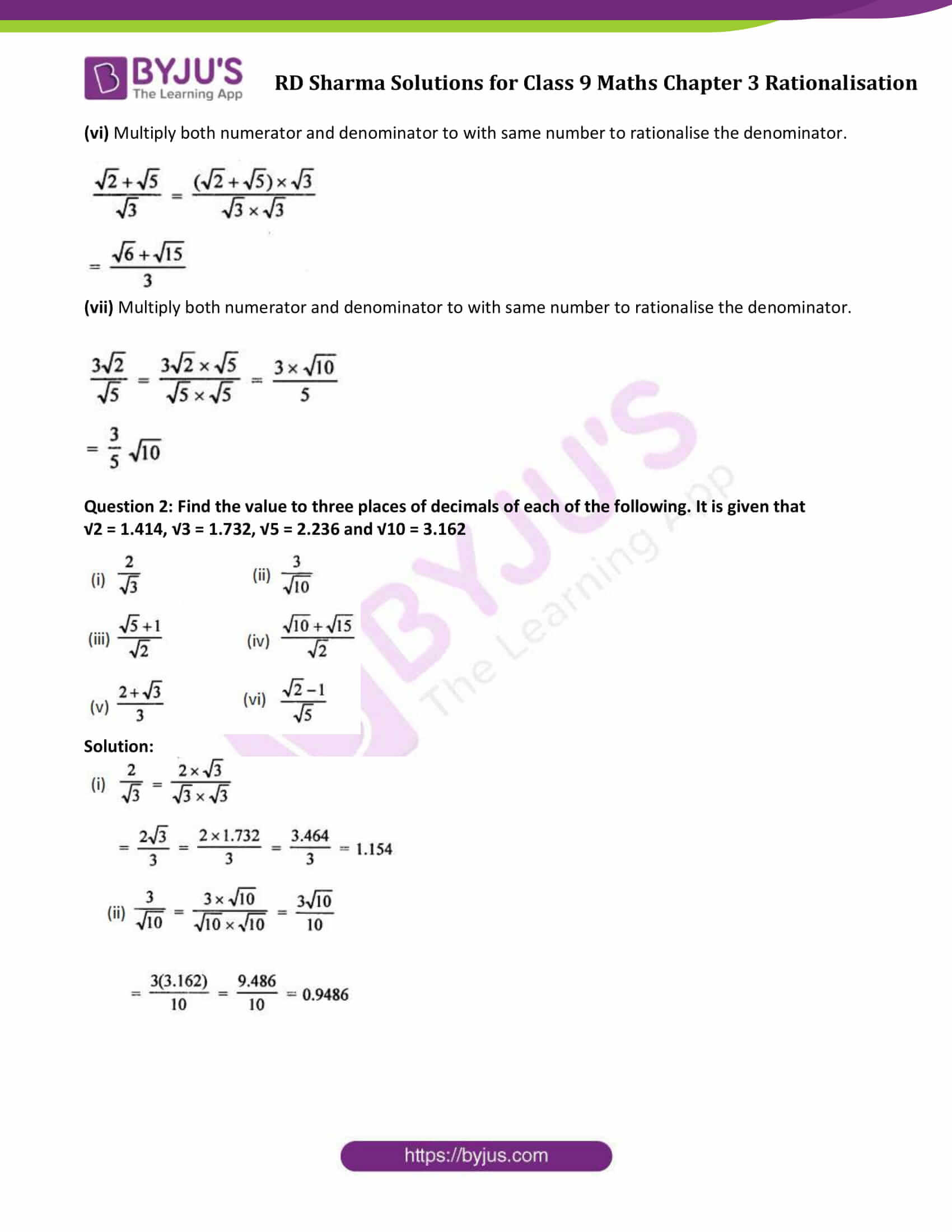
(vi) Multiply both the numerator and denominator with the same number to rationalise the denominator.

(vii) Multiply both the numerator and denominator with the same number to rationalise the denominator.

Question 2: Find the value to three places of decimals of each of the following. It is given that

√2 = 1.414, √3 = 1.732, √5 = 2.236 and √10 = 3.162

Solution:

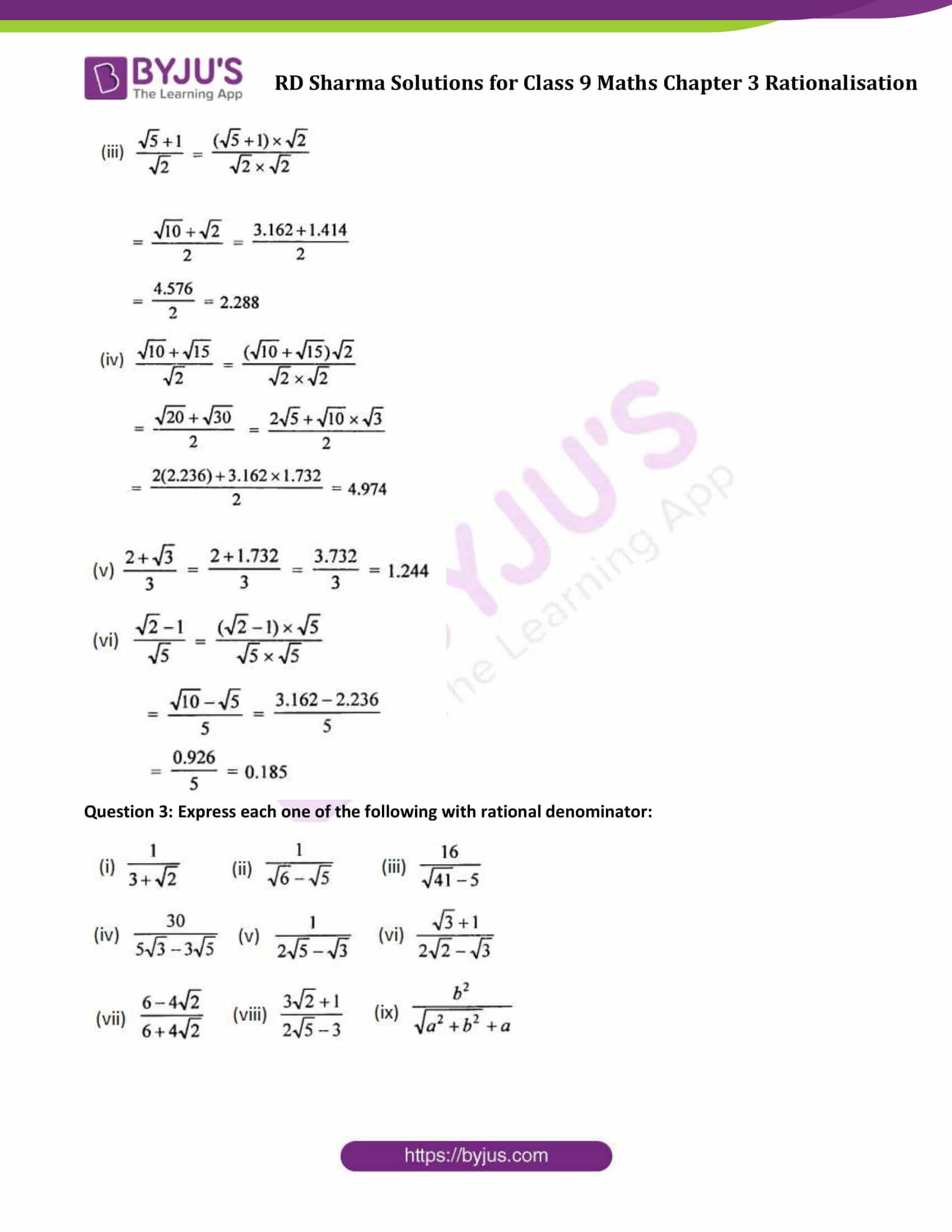
Question 3: Express each one of the following with a rational denominator:

Solution:

Using identity: (a + b) (a – b) = a² – b²

(i) Multiply and divide the given number by 3−√2

(ii) Multiply and divide the given number by √6 + √5

(iii) Multiply and divide the given number by √41 + 5

(iv) Multiply and divide the given number by 5√3 + 3√5

(v) Multiply and divide the given number by 2√5 + √3

(vi) Multiply and divide the given number by 2√2 + √3

(vii) Multiply and divide the given number by 6 – 4√2

(viii) Multiply and divide the given number by 2√5 + 3

(ix) Multiply and divide the given number by √(a²+b²) – a

Question 4: Rationales the denominator and simplify:

Solution:

[Use identities: (a + b) (a – b) = a² – b² ; (a + b)² = (a² + 2ab + b² and (a – b)² = (a² – 2ab + b² ]

(i) Multiply both numerator and denominator by √3–√2 to rationalise the denominator.

(ii) Multiply both numerator and denominator by 7–4√3 to rationalise the denominator.

(iii) Multiply both numerator and denominator by 3+2√2 to rationalise the denominator.

(iv) Multiply both numerator and denominator by 3√5+2√6 to rationalise the denominator.

(v) Multiply both numerator and denominator by √48–√18 to rationalise the denominator.

(vi) Multiply both numerator and denominator by 2√2 – 3√3 to rationalise the denominator.

Exercise VSAQs

Question 1: Write the value of (2 + √3) (2 – √3).

Solution:

(2 + √3) (2 – √3)

= (2)² – (√3)²

= 4 – 3

= 1

Question 2: Write the reciprocal of 5 + √2.

Solution:

Question 3: Write the rationalisation factor of 7 – 3√5.

Solution:

The rationalisation factor of 7 – 3√5 is 7 + 3√5

Question 4: If

Find the values of x and y.

Solution:

[Using identities : (a + b)(a – b) = a² – b² and (a – b)² = a² + b² – 2ab]

Question 5: If x = √2 – 1, then write the value of 1/x.

Solution:

x = √2 – 1

or 1/x = 1/(√2 – 1)

Rationalising denominator, we have

= 1/(√2 – 1) x (√2 + 1)/(√2 + 1)

= (√2 + 1)/(2-1)

= √2 + 1

Question 6: Simplify

Solution:

[ Because: (a + b)² = a² + b² + 2ab ]

Question 7: Simplify

Solution:

[ Because: (a – b)² = a² + b² – 2ab ]

Question 8: If a = √2 +1, then find the value of a – 1/a.

Solution:

Given: a = √2 + 1

1/a = 1/(√2 + 1)

= 1/(√2 + 1) x (√2 – 1)/(√2 – 1)

= (√2 – 1)/ ((√2)² – (1)²)

= (√2 – 1)/1

= √2 – 1

Now,

a – 1/a = (√2 + 1) – (√2 – 1)

= 2

Question 9: If x = 2 + √3, find the value of x + 1/x.

Solution:

Given: x = 2 + √3

1/x = 1/(2 + √3)

= 1/(2 + √3) x (2 – √3)/(2 – √3)

= (2 – √3)/ ((2)² – (√3)²)

= (2 – √3)/(4-3)

= (2 – √3)

Now,

x + 1/x = (2 + √3) + (2 – √3)

= 4

Question 10: Write the rationalisation factor of √5 – 2.

Solution:

The rationalisation factor of √5 – 2 is √5 + 2

Question 11: If x = 3 + 2√2, then find the value of √x – 1/√x.

Solution:

RD Sharma Solutions for Class 9 Maths Chapter 3 Rationalisation

In Chapter 3 of Class 9 RD Sharma Solutions, students will study important concepts on rationalisation as listed below:

• Rationalisation introduction
• Rationalisation of the denominator
• Some important algebraic identities

Students can get acquainted with various methods of solving problems with ease and face exams more confidently by practising RD Sharma Solutions for Class 9 Maths Chapter 3 Rationalisation. Hence, students are suggested to solve these solutions without fail to procure high marks in the annual exams.