RD Sharma Solutions for Class 9 Mathematics Chapter 10 Exercise 10.5 Congruent Triangles are provided in this article. Here, students will learn about the congruence criterion of right triangles, mainly using RHS (Right-angle-Hypotenuse-Side) and its related concepts in an interesting and interactive way. Now, this topic can easily be learnt with RD Sharma Solutions for Class 9 with step-by-step illustrations. Download the solutions in PDF for free from the links provided below.
Download PDF of RD Sharma Solutions for Class 9 Maths Chapter 10 Congruent Triangles Exercise 10.5
Access Answers to D Sharma Solutions for Class 9 Maths Chapter 10 Congruent Triangles Exercise 10.5 Page Number 10.51
Question 1: ABC is a triangle, and D is the mid-point of BC. The perpendiculars from D to AB and AC are equal. Prove that the triangle is isosceles.
Solution:
Given: D is the midpoint of BC and PD = DQ in a triangle ABC.
To prove: ABC is isosceles triangle.

In △BDP and △CDQ
PD = QD (Given)
BD = DC (D is mid-point)
∠BPD = ∠CQD = 90o
By RHS Criterion: △BDP ≅ △CDQ
BP = CQ … (i) (By CPCT)
In △APD and △AQD
PD = QD (given)
AD = AD (common)
APD = AQD = 90 o
By RHS Criterion: △APD ≅ △AQD
So, PA = QA … (ii) (By CPCT)
Adding (i) and (ii)
BP + PA = CQ + QA
AB = AC
Two sides of the triangle are equal, so ABC is an isosceles.
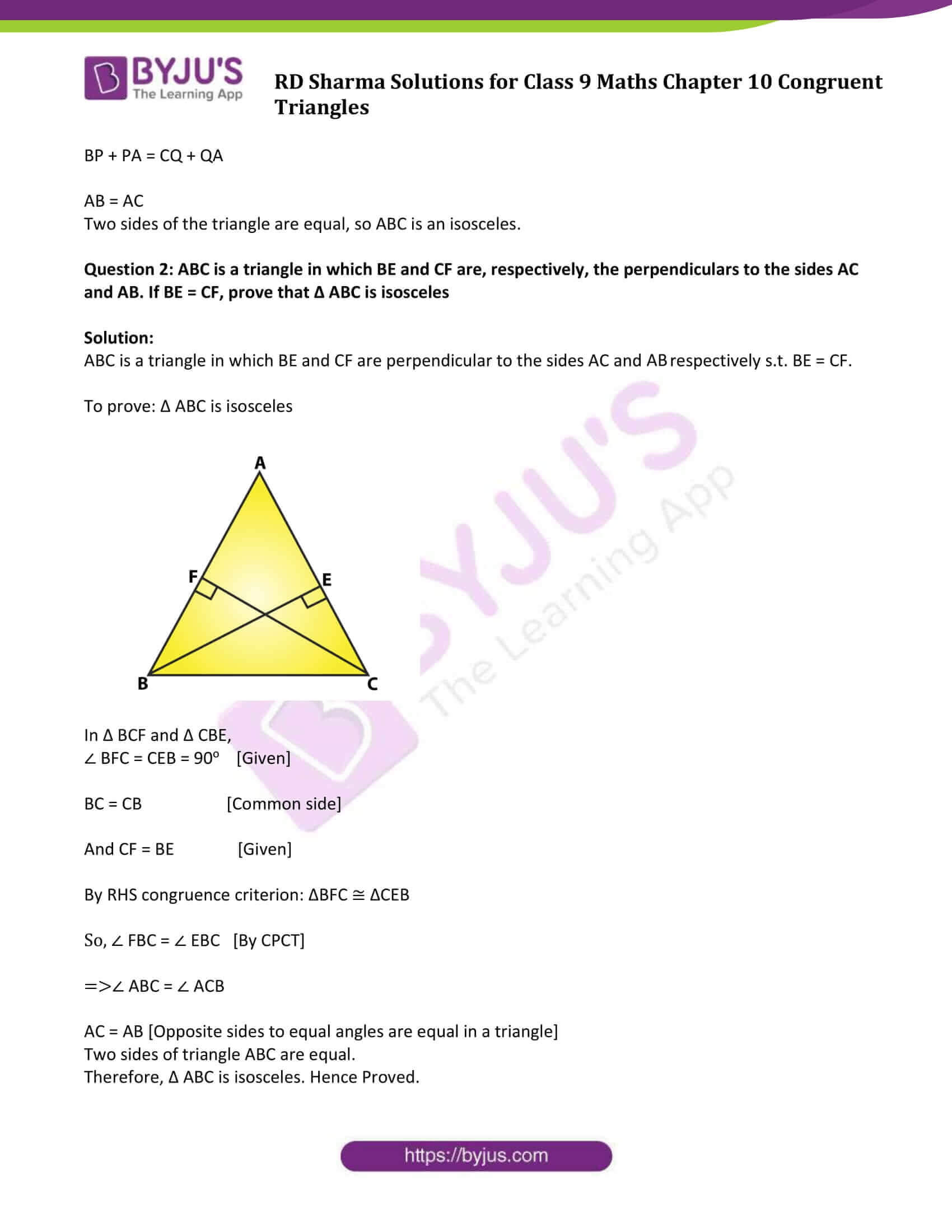
Question 2: ABC is a triangle in which BE and CF are, respectively, the perpendiculars to the sides AC and AB. If BE = CF, prove that Δ ABC is isosceles
Solution:
ABC is a triangle in which BE and CF are perpendicular to the sides AC and AB, respectively, s.t. BE = CF.
To prove: Δ ABC is isosceles

In Δ BCF and Δ CBE,
∠ BFC = CEB = 90o [Given]
BC = CB [Common side]
And CF = BE [Given]
By RHS congruence criterion: ΔBFC ≅ ΔCEB
So, ∠ FBC = ∠ EBC [By CPCT]
⇒∠ ABC = ∠ ACB
AC = AB [Opposite sides to equal angles are equal in a triangle]
Two sides of triangle ABC are equal.
Therefore, Δ ABC is isosceles. Hence Proved.
Question 3: If perpendiculars from any point within an angle on its arms are congruent. Prove that it lies on the bisector of that angle.
Solution:

Consider an angle ABC and BP be one of the arms within the angle.
Draw perpendiculars PN and PM on the arms BC and BA.
In Δ BPM and Δ BPN,
∠ BMP = ∠ BNP = 90° [given]
BP = BP [Common side]
MP = NP [given]
By RHS congruence criterion: ΔBPM≅ΔBPN
So, ∠ MBP = ∠ NBP [ By CPCT]
BP is the angular bisector of ∠ABC.
Hence proved
RD Sharma Solutions for Class 9 Maths Chapter 10 Congruent Triangles Exercise 10.5
RD Sharma Solutions Class 9 Maths Chapter 10 Congruent Triangles Exercise 10.5 is based on the topic Congruence Criterion – Right-angle- Hypotenuse-Side. According to the criteria, two triangles are congruent if the hypotenuse and one side of one triangle are respectively equal to the hypotenuse and one side of the other triangle.


Comments